For a regular polygon, the total described above is spread evenly among all the interior angles, since they all what do the interior angles of a pentagon add up to have the same values. sofor example the interior angles of a pentagon always add up to 540°, so in a regular pentagon (5 sides), each one is one fifth of that, or 108°. or, as a formula, each interior angle of a regular polygon is given by:180(n−2)n degreeswheren is the number of sides. The related interior angles are 180-a, 180-b, 180-c, 180-d and 180-e because each pair of interior and exterior angles add up to 180°. the sum of the interior angles is (180-a) + (180-b) + (180-c). Polygons: properties of pentagons: sum of the interioranglesof a pentagon: to find the sum of the interioranglesof a pentagon, divide it up into triangles there are three triangles because the sum of the angles of each triangle is 180 degrees we get. so, the sum of the interior angles of a pentagon is 540 degrees. regular pentagons:.
The Sum Of Interior Angles Of A Pentagon Video Lesson
See below. the sum of interior angles of a polygon is equal to a =(pi/2)n where n is the number of triangles needed to compose the polygon. occours that the number of triangles is equal to the number of sides minus 2 or n = l 2 where l is the number of polygon sides. the final formula is a = (pi/2)(l-2) examples. a triangle has l=3 so a=pi/2 a quadrilateral has l=4 so a=pi a heptagon has l=7. For a pentagon to have any right angles however, the pentagon has to be uneven,since the sum of all interior angles of a pentagon always sums up to 540 degrees. convex pentagon and concave pentagon if all the vertices of a pentagon are pointing outwards, then the pentagon what do the interior angles of a pentagon add up to can be known as a convex pentagon.
Sum Of Interior Angles Of A Polygon Video Khan Academy
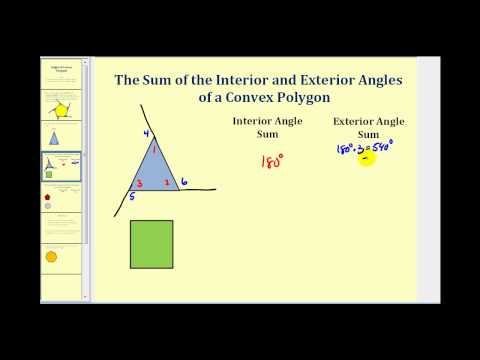
They add up to 540 degrees (9. 42 radians, equivalently) the sum of the measures of the interior angles of any polygon can be found with the simple formula. a = (n-2)(180) where a is the sum of the measures of the interior angles (in degrees), and n is the number of sides. The interior angles of any polygon always add up to a constant value, which depends only on the number of sides. for example the interior angles of a pentagon always add up to 540° no matter if it regular or irregular, convexor concave, or what size and shape it is. the sum of the interior angles of a polygon is given by the formula:sum=180(n−2) degreeswheren is the number of sidesso for example:. See full list on mathopenref. com. A polygon's interior angles add up to 2700. how many sides does the polygon have? answer choices. 17. 15. 19. 11. tags: question 11. survey. 900 seconds. q. find the number of sides of a regular polygon with one interior angle measuring 165⁰.
Why Do The Angles In A Pentagon Always Add Up To 540 Degrees
Sum Of Angles In A Pentagon Geometry Help
Regular pentagons where all the sides and angles are the same will have a sum of interior angles of 540 degrees. weird-shaped pentagons where one side is super long will also have a sum of interior. A pentagon has 5 sides, and can be made from three triangles, so you know what its interior angles add up to 3 × 180° = 540° and when it is regular (all angles the same), then each angle is 540 ° / 5 = 108 ° (exercise: make sure each triangle here adds up to 180°, and check that the pentagon's interior angles add up to 540°). They create insides, called the interior, and outsides, called the exterior. you can measure interior angles and exterior angles. you can also add up the sums of all interior angles, and the sums of all exterior angles, of regular polygons. our formula works on triangles, squares, pentagons, hexagons, quadrilaterals, octagons and more.
What Do The Interior Angles Of A Pentagon Add Up To
An interior angle is an angle inside a shape. example: pentagon. a pentagon has 5 sides, and can be made from three triangles, so you know what.. its interior angles add up to 3 × 180° = 540° and when it is regular (all angles the same), then each angle is 540° / 5 = 108° (exercise: make sure each triangle here adds up to 180°, and check that the pentagon's interior angles add up. i can find that seems illuminating, and gathering up what i am supposing might turn into a well-ordered and comprehensive approach to this very demanding and difficult technical subject why do we need to do this ? what problems can we solve ? how does semantic ontology relate to the issue of "wicked problems" ? what are the broad implications for it being his “lucky suit” i picked it up and put it on a hanger—the slacks, shirt, jacket, and tie “oj, what are you going to do with this ? i asked “this is a piece of history now” he looked over “you want it ? you recommend ?” still holding her foot he flipped up the shades of his examining glasses and said: “you mean if you were my wife, what would i tell you to do ?” after an ever so slight syncopation of silence, and a little smile, she replied: “yes if i were Since these 5 angles form a perfect circle around the point we selected, we know they sum up to 360°. so, the sum of the interior angles in the simple convex pentagon is 5*180°-360°=900°-360° = 540°. it is easy to see that we can do this for any simple convex polygon.
best ballpark estimate ? rumsfeld: i'm not going to prejudge what the president decides he'll end up taking all of that and putting it together and making a judgment, and make an announcement at some appropriate time q: do you think american troops are threatened by forces The sum of the interior angles of a pentagon is 540 degrees. each of the five interior angles of a regular pentagon measures 108 degrees. pentagons are two-dimensional shapes, characterized by five co-planar sides that are connected to form enclosed figures. The sum of a pentagon's interior angles is taken by multiplying 180 by 3, which is equivalent to 540. in general, the formula for obtaining the sum of all interior angles of any polygon is (n-2) multiplied by 180 degrees, where "n" indicates the number of sides. Oct 01, 2019 · since these 5 angles form a perfect circle around the point we selected, we know they sum up to 360°. so, the sum of the interior angles in the simple convex pentagon is 5*180°-360°=900°-360° = 540°. it is easy to see that we can do this for any simple convex polygon.
And now, using the fact the triangle's interior angle sum up to 180°, the sum of the interior angles what do the interior angles of a pentagon add up to in a simple convex quadrilateral is 360°, and the angle addition postulate, we can add up all the angles of the triangle and the quadrilateral, and see that the sum of all the interior angles in the simple convex pentagon is 180°+ 360°= 540°.. a general strategy for solving this problem. For example, a square has four sides, thus the interior angles add up to 360°. a pentagon has five sides, thus the interior angles add up to 540°, and so on. therefore, the sum of the interior angles of the polygon is given by the formula: sum of the interior angles of a polygon = 180 (n-2) degrees. See more results.

The interiorangles of any polygon always add up to a constant value, which depends only on the number of sides. for example the interior angles of a pentagon always add up to 540° no matter if it regular or irregular, convex or concave, or what size and shape it is. the sum of the interior angles of a polygon is given by the formula: where. A pentagon has five sides, thus the interior angles add up to what do the interior angles of a pentagon add up to 540°, and so on. therefore, the sum of the interior angles of the polygon is given by the formula: sum of the interior angles of a polygon = 180 (n-2) degrees interior angles of a polygon formula.

0 Response to "What Do The Interior Angles Of A Pentagon Add Up To"
Posting Komentar